Direct link: https://www.geogebra.org/m/u2m3gnzj
The above is a GeoGebra applet that can be customised for any energy problem. Simply make a copy of it and change the values or labels as needed. This can be integrated into either GeoGebra Classroom or Google Classroom (as a GeoGebra assignment) and the teacher can then monitor every student’s attempt at interpreting the energy changes in the problem. The teacher can also choose different extents of scaffolding, e.g. provide the initial or final states and ask students to fill in the rest.
What is an LOL Diagram?
An LOL diagram is a tool used to visualize and analyze the conservation of energy in physical systems. “LOL” does not stand for anything meaningful. Rather, they just form the shapes of the two sets of axes and the circle in between. They help clarify which objects or components are included in the energy system being considered and how energy is transferred or transformed within that system.
In LOL diagrams:
- An energy system is defined as an object or a collection of objects whose energies are being tracked.
- LOL diagrams consist of three parts: a L-shaped bar-chart representing the initial state, an O representing the object (or system) of interest and another L-shaped bar-chart representing the final state.
- There can also be energy transferred into the system or out of the system if the system is not closed or isolated. These are represented using horizontal bars below the L axes, with arrows indicating if they are energy transferred in or out.
When performing calculations involving the initial and final energy states, the energy transferred into the system is added to the initial energy state while the energy transferred out of the system is added to the final energy state. The sums must be equal. In other words,
Initial energy stores + Energy transferred into system = Final energy stores + Energy transferred out of system
How do I use an LOL diagram?
Here’s a breakdown of how LOL diagrams are used, using an example of a falling mass:
- System Definition (O):
- Choose what is part of the energy system (objects whose energies are being tracked) and what isn’t.
- For example, in the case of a falling mass, the mass itself and the Earth are part of the energy system.
- Initial State (L):
- Represent the initial energy configuration of the system.
- Identify the types of energy present in the system at the beginning. In this example, we begin with some gravitational potential energy.
- Transition:
- Show how energy changes as the system evolves. In the falling mass example, the gravitational potential energy decreases, and kinetic energy increases.
- Final State (L):
- Represent the energy distribution in the system at the end of the process.
- In the falling mass example, at the point just before it hits the ground, kinetic energy is maximized, and gravitational potential energy is minimized.
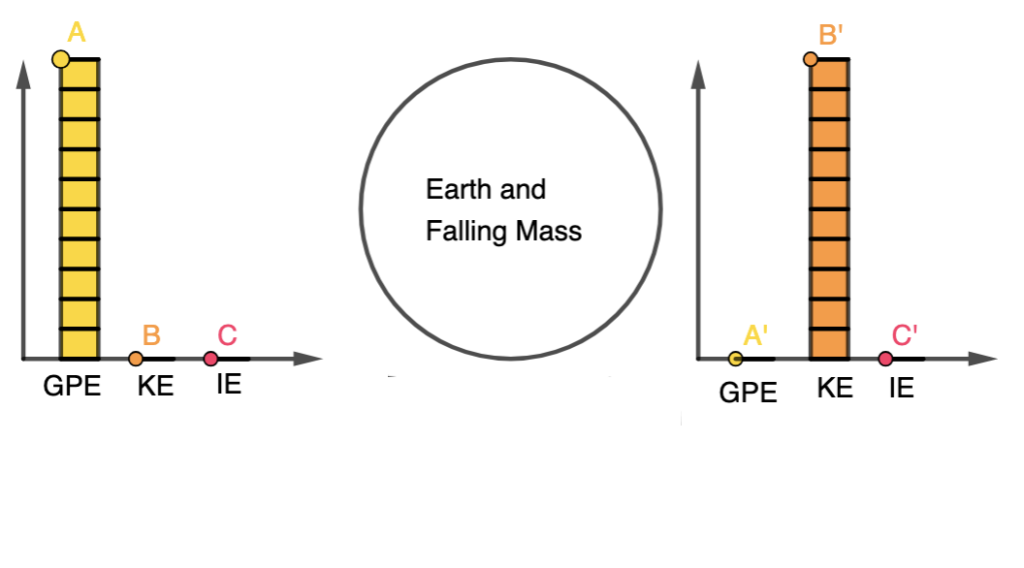
LOL diagrams illustrate that energy within the system is conserved, meaning the total energy in the system remains constant.
External work (work done by forces outside the defined system) may impact the system’s energy, but internal work (work done within the defined system) does not change the total energy of the system.
The mathematical representation of the above problem will then simply be:
GPE = KE
$mgh = \dfrac{1}{2}mv^2$
This problem seems a bit trivial. Since LOL diagrams are a visual tool to help students and scientists analyze energy transformations and conservation, they can be used for making it easier to set up and solve conservation of energy equations in problems of greater complexity.
LOL Diagram of an Electrical Circuit
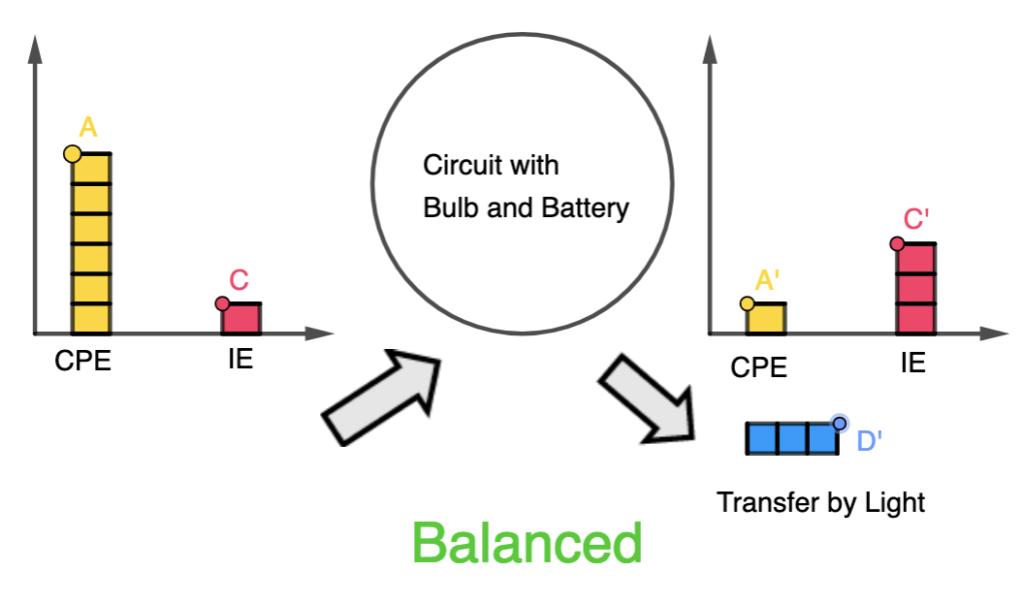
It is also important to note that the choice of the object (or system) of interest will result in different LOL diagrams for the same phenomenon.
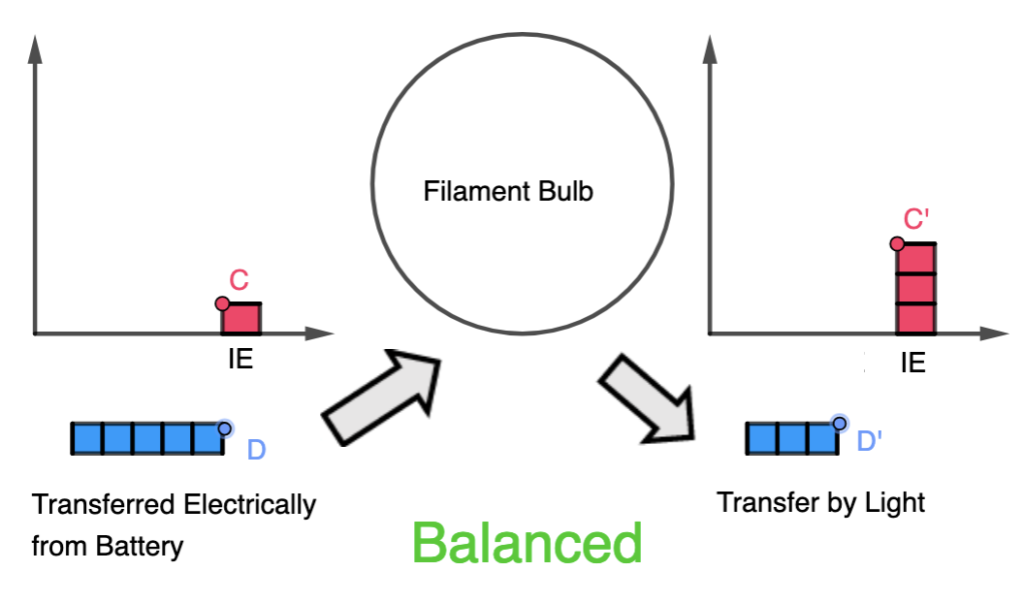
For example, consider a filament bulb in a circuit with a battery. The system at room temperature also has some energy in the internal store (or internal energy, which consists of the kinetic and potential energies of the particles in the system).
When considering the filament as the object of interest, when energy is transferred electrically from the battery, part of it is transferred by light from the bulb to the surroundings and another part is added to the internal store, as it heats up the filament light.
On the other hand, when considering the circuit as the whole, the chemical potential store of the battery is included in the initial energy state of the system. Hence, there is no additional energy transfer into the system but the energy transfer output is still the same.
How do I modify the GeoGebra applet to make my own LOL Diagram?
Here’s a video that demonstrates how the editing process is done, in a little more than one minute!
The final product is here.