This GeoGebra applet was modified from an existing applet to show the relationship between the pressure-distance and displacement-distance graph of a progressive longitudinal wave.
A physics demonstration on how to measure the speed of sound in air using Audacity, an open source audio recording software. There are Windows and Mac versions of this free software, and even a portable version that can run off a flash drive without needing to be installed on a computer (for school systems with stricter measures regarding installing of software).
The sound is reflected along a long hollow tube that somehow, existed in our school’s laboratory. The two sound signals were picked up using a clip-on microphone attached to the open end of the tube and plugged into the laptop. I used my son’s castanet which gives a crisp sound and hence, a simple waveform that will not have the echo overlapping with the generated sound. The timing at which the sound signals were first detected were read and subtracted to obtain the time taken for the wave to travel up and down the 237 cm tube.
The value of the speed of sound calculated is 356 m/s, which is a bit on the high side due to the temperature of 35°C and relative humidity of between 60-95% when the reading was carried out.
If you are interested, you can check out how the software can be used to determine the frequency of a tuning fork.
Audacity is a free audio recording and editing software downloadable from http://audacity.sourceforge.net. It is cross-platform meaning you can run it on PC or Mac.
Once you have the software installed, you can try out some of its simpler functions, such as recording and playing back. You can also generate tones of known frequencies, which will be useful for experiments such as using stationary waves to determine the speed of sound in air.
Frequency and pitch
The first activity serves as an introduction to the software and can be easily carried out. All you need is a computer with Audacity installed and a microphone connected to it. Tuning forks of different frequencies will be best for this activity because of the simple and pure waveforms generated. A regular wave pattern can also be recorded with the help of a musical instrument such as a guitar or by singing a note.
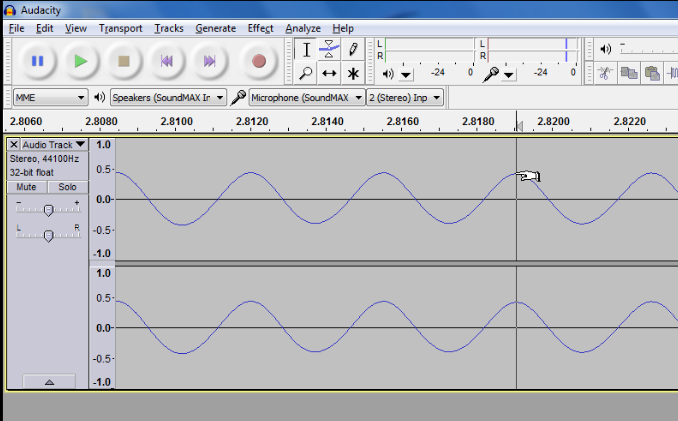
To make a sound with the tuning fork, strike it against something hard such as the heel of your hand. The two prongs of the fork, known as “tines,” will then vibrate with a fixed frequency, thus generating a waveform with a displacement that is almost sinusoidal. Place this tuning fork next to the microphone and you should see a densely packed waveform like the following:
Zoom into the peaks recorded by clicking on the peaks of interest and pressing Ctrl 1 for Windows or Command 1 for Mac. You should observe a repeated pattern in the waveform.
By reading off the time difference on the horizontal axis between two peaks, you can measure the period of the wave. Using $$f=\frac{1}{T}$$ where f is the frequency of the wave, and T is the period, you can verify the frequency measured with the known value of the tuning fork’s frequency.
In the above graph, the period is 2.8190-2.8155 = 0.0035s. The frequency of the tuning fork used is given by $$f=\frac{1}{T}=\frac{1}{0.0035}= 286 \text{ Hz}$$ which is roughly that of a D note.